Average Value Theorem
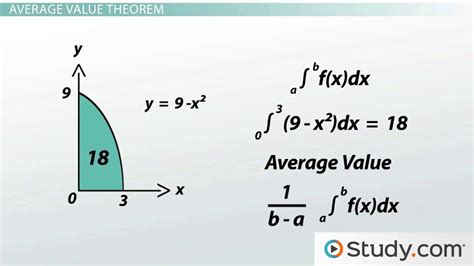
The Average Value Theorem (AVT) is a fundamental concept in calculus, specifically in the realm of integral calculus. It states that if a function f(x) is continuous on a closed interval [a, b], then there exists a point c in the interval (a, b) such that the average value of the function over the interval is equal to the value of the function at c. This theorem has far-reaching implications in various fields, including physics, engineering, and economics, and is a crucial tool for mathematicians and scientists alike.
To understand the Average Value Theorem, it's essential to grasp the concept of average value. The average value of a function f(x) over an interval [a, b] is defined as the definite integral of the function over the interval, divided by the length of the interval. Mathematically, this can be represented as: (1/(b-a)) * ∫[a, b] f(x) dx. The AVT states that this average value is equal to the value of the function at some point c in the interval (a, b), i.e., f(c) = (1/(b-a)) * ∫[a, b] f(x) dx.
Mathematical Formulation and Proof

The Average Value Theorem can be formulated mathematically as follows: Let f(x) be a continuous function on the closed interval [a, b]. Then, there exists a point c in the interval (a, b) such that ∫[a, b] f(x) dx = f(c) * (b-a). The proof of this theorem involves the application of the Extreme Value Theorem and the Mean Value Theorem. The Extreme Value Theorem states that a continuous function on a closed interval attains its maximum and minimum values, while the Mean Value Theorem states that a differentiable function on an open interval has a point where the derivative is equal to the average rate of change.
The proof of the AVT proceeds by considering the function F(x) = ∫[a, x] f(t) dt, which is continuous on [a, b] and differentiable on (a, b). By the Mean Value Theorem, there exists a point c in (a, b) such that F'(c) = (F(b) - F(a)) / (b-a). Since F'(x) = f(x), we have f(c) = (F(b) - F(a)) / (b-a) = (∫[a, b] f(x) dx) / (b-a), which establishes the AVT.
Geometric Interpretation and Applications
The Average Value Theorem has a geometric interpretation, which provides valuable insight into its significance. The theorem states that the area under the curve of a function f(x) over an interval [a, b] is equal to the area of a rectangle with height f(c) and width b-a. This geometric interpretation has numerous applications in physics, engineering, and economics. For instance, in physics, the AVT is used to calculate the average velocity of an object over a given time interval, while in economics, it is used to determine the average cost of production over a given period.
| Application | Description |
|---|---|
| Physics | Calculating average velocity and acceleration |
| Engineering | Designing systems with optimal performance |
| Economics | Determining average cost of production and revenue |
Key Points
- The Average Value Theorem states that a continuous function on a closed interval has a point where the average value is equal to the function value.
- The theorem has a geometric interpretation, where the area under the curve is equal to the area of a rectangle.
- The AVT has numerous applications in physics, engineering, and economics, including calculating average velocity and acceleration, designing systems with optimal performance, and determining average cost of production and revenue.
- The theorem is proved using the Extreme Value Theorem and the Mean Value Theorem.
- The AVT is a fundamental concept in calculus, with far-reaching implications in various fields.
Implications and Extensions
The Average Value Theorem has significant implications for the study of functions and their behavior. It provides a powerful tool for analyzing functions and understanding their properties over a given interval. The theorem also has extensions and generalizations, such as the Mean Value Theorem for Integrals, which states that a continuous function on a closed interval has a point where the average value is equal to the function value, and the Cauchy Mean Value Theorem, which provides a more general version of the Mean Value Theorem.
The AVT also has connections to other areas of mathematics, such as measure theory and functional analysis. In measure theory, the theorem is used to define the average value of a function with respect to a measure, while in functional analysis, it is used to study the properties of linear operators and their averages.
Open Problems and Future Research
Despite its significance and widespread applications, the Average Value Theorem remains an active area of research, with many open problems and potential extensions. One area of ongoing research is the development of new proofs and generalizations of the theorem, such as the use of non-standard analysis and the study of the theorem in non-Euclidean spaces. Another area of research is the application of the AVT to new fields, such as data analysis and machine learning, where the theorem can be used to develop new algorithms and models.
What is the Average Value Theorem?
+The Average Value Theorem states that a continuous function on a closed interval has a point where the average value is equal to the function value.
What are the applications of the Average Value Theorem?
+The Average Value Theorem has numerous applications in physics, engineering, and economics, including calculating average velocity and acceleration, designing systems with optimal performance, and determining average cost of production and revenue.
How is the Average Value Theorem proved?
+The Average Value Theorem is proved using the Extreme Value Theorem and the Mean Value Theorem.