Convert Mixed Numbers To Improper Fractions

Converting mixed numbers to improper fractions is a fundamental concept in mathematics, particularly in the realm of fractions. A mixed number is a combination of a whole number and a proper fraction, where the whole number represents the number of whole units and the fraction represents the remaining part of the unit. On the other hand, an improper fraction is a fraction where the numerator is greater than or equal to the denominator, representing a value greater than or equal to one whole unit.
Understanding Mixed Numbers and Improper Fractions

To grasp the conversion process, it’s essential to understand the structure of both mixed numbers and improper fractions. A mixed number is typically represented as a \frac{b}{c}, where a is the whole number part, and \frac{b}{c} is the fractional part. An improper fraction, however, is represented as \frac{d}{e}, where d is the numerator, and e is the denominator.
Conversion Process
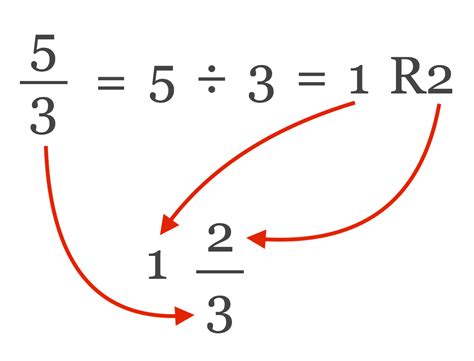
The process of converting a mixed number to an improper fraction involves a simple mathematical operation. Given a mixed number a \frac{b}{c}, the equivalent improper fraction can be found by multiplying the whole number part a by the denominator c and then adding the numerator b. This sum becomes the new numerator, while the denominator remains unchanged. The formula for this conversion is \frac{(a \times c) + b}{c}.
| Mixed Number | Improper Fraction |
|---|---|
| $2 \frac{3}{4}$ | $\frac{(2 \times 4) + 3}{4} = \frac{8 + 3}{4} = \frac{11}{4}$ |
| $1 \frac{1}{2}$ | $\frac{(1 \times 2) + 1}{2} = \frac{2 + 1}{2} = \frac{3}{2}$ |

Practical Applications and Examples
The conversion between mixed numbers and improper fractions has numerous practical applications in real-world scenarios, such as cooking, construction, and financial calculations. For instance, a recipe might call for 2 \frac{1}{4} cups of flour, which can be represented as \frac{9}{4} cups in improper fraction form, facilitating easier multiplication or division in scaling the recipe.
Technical Specifications and Considerations
When dealing with mixed numbers and improper fractions in technical or professional contexts, it’s essential to consider the specific requirements or conventions of the field. For example, in engineering or architectural designs, precise measurements are critical, and the ability to convert between different forms of fractions can be invaluable. Understanding the equivalent values in different forms can help in avoiding errors and ensuring accuracy in calculations.
Key Points
- Mixed numbers combine whole numbers and proper fractions, representing values greater than one whole unit.
- Improper fractions have numerators greater than or equal to their denominators, representing values greater than or equal to one whole unit.
- The conversion from a mixed number to an improper fraction involves multiplying the whole number part by the denominator, adding the numerator, and keeping the denominator the same.
- This conversion is crucial for various mathematical operations and has practical applications in real-world scenarios.
- Understanding and accurately converting between mixed numbers and improper fractions can prevent errors and ensure precision in calculations.
In conclusion, converting mixed numbers to improper fractions is a straightforward yet critical process in mathematics. By understanding the structure of both forms and applying the conversion formula, individuals can easily switch between these representations, facilitating a wide range of mathematical operations and real-world applications. This skill not only enhances mathematical fluency but also contributes to accuracy and efficiency in problem-solving across various disciplines.
What is the primary difference between a mixed number and an improper fraction?
+A mixed number combines a whole number and a proper fraction, while an improper fraction has a numerator that is greater than or equal to its denominator, representing a value greater than or equal to one whole unit.
How do you convert a mixed number to an improper fraction?
+You convert a mixed number to an improper fraction by multiplying the whole number part by the denominator, adding the numerator, and keeping the denominator the same. The formula is \frac{(a \times c) + b}{c} for a mixed number a \frac{b}{c}.
What are some practical applications of converting mixed numbers to improper fractions?
+Practical applications include cooking, where scaling recipes requires precise measurements; construction, where exact dimensions are crucial; and financial calculations, where accuracy is paramount.
Related Terms:
- Improper fraction calculator
- Fraction to mixed number
- Mixed fraction
- Improper fraction to proper fraction
- Mixed number calculator