Factors Of 75

The concept of factors is a fundamental aspect of mathematics, particularly in the realm of number theory. Factors of a number are the numbers that can be multiplied together to get that number. In this article, we will delve into the factors of 75, exploring what they are, how to find them, and their significance in mathematical operations.
What are the Factors of 75?
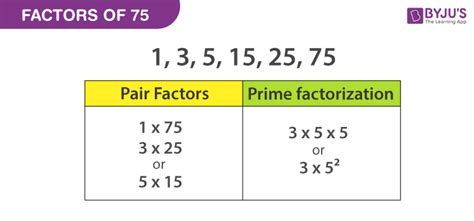
To find the factors of 75, we need to identify all the numbers that divide 75 without leaving a remainder. This involves breaking down 75 into its prime factors, which are the prime numbers that multiply together to give 75. The prime factorization of 75 is 3 * 5 * 5, or (3 \times 5^2). From this prime factorization, we can derive all the factors of 75 by combining the prime factors in all possible ways.
Listing the Factors of 75
The factors of 75 are derived from the combinations of its prime factors, which are 3 and 5. The combinations include using none of a particular prime factor (which equates to using 1, since (3^0 = 1) and (5^0 = 1)), using one instance of a prime factor, and using all instances of a prime factor. Therefore, the factors are: 1, 3, 5, 15, 25, and 75. These numbers are the factors because they can all divide 75 without leaving a remainder.
| Factor | Prime Factorization | Multiplication to 75 |
|---|---|---|
| 1 | 3^0 \times 5^0 | 1 \times 75 = 75 |
| 3 | 3^1 \times 5^0 | 3 \times 25 = 75 |
| 5 | 3^0 \times 5^1 | 5 \times 15 = 75 |
| 15 | 3^1 \times 5^1 | 15 \times 5 = 75 |
| 25 | 3^0 \times 5^2 | 25 \times 3 = 75 |
| 75 | 3^1 \times 5^2 | 75 \times 1 = 75 |

Key Points
- The factors of 75 are 1, 3, 5, 15, 25, and 75.
- The prime factorization of 75 is 3 \times 5^2.
- Factors are essential in mathematics for understanding divisibility, simplifying fractions, and solving algebraic equations.
- Prime factorization is a key method for finding all the factors of a number.
- Understanding factors and prime factorization can simplify various mathematical operations and provide insights into number theory.
Significance of Factors in Mathematics

Beyond just listing the factors of 75, understanding the concept of factors has broader implications in mathematics and real-world applications. Factors are crucial in simplifying fractions, where dividing both the numerator and denominator by their greatest common factor (GCF) simplifies the fraction to its lowest terms. They are also essential in solving algebraic equations, particularly in finding the roots of quadratic equations or simplifying expressions.
Real-World Applications
The application of factors extends beyond the realm of pure mathematics into practical, real-world scenarios. For instance, in construction or design, understanding the factors of a number can help in determining the dimensions of rooms, the layout of tiles, or the arrangement of materials in a way that minimizes waste and optimizes space. In finance, factors can be used to calculate interest rates, understand compound interest, and make informed investment decisions.
What is the importance of prime factorization in finding factors?
+Prime factorization is crucial because it breaks down a number into its fundamental building blocks, making it easier to find all possible factors by combining these prime factors in different ways.
How do factors relate to divisibility rules?
+Factors directly relate to divisibility rules because if a number is divisible by another, it means the divisor is a factor of the number. Understanding factors can help in applying divisibility rules to check if a number can be divided evenly by another.
Can factors be used in fractions and algebra?
+Yes, factors are essential in simplifying fractions by dividing both the numerator and denominator by their greatest common factor. In algebra, factors are used to solve equations and simplify expressions by factoring out common factors.
In conclusion, the factors of 75, which are 1, 3, 5, 15, 25, and 75, provide a foundational understanding of the number’s properties and its relationship to other numbers. The method of finding these factors through prime factorization is a powerful tool in mathematics, applicable not only to the number 75 but to all numbers. This understanding has profound implications for various mathematical operations and real-world applications, demonstrating the interconnectedness and beauty of mathematics.



