How To Determine Whether A Function Is Even Or Odd

Determining whether a function is even or odd is a fundamental concept in mathematics, particularly in algebra and calculus. Even and odd functions have distinct properties that make them useful in various mathematical and real-world applications. In this article, we will explore the definitions, characteristics, and examples of even and odd functions, as well as provide a step-by-step guide on how to determine the parity of a function.
Key Points
- A function is even if f(x) = f(-x) for all x in its domain.
- A function is odd if f(-x) = -f(x) for all x in its domain.
- Even functions are symmetric with respect to the y-axis, while odd functions are symmetric with respect to the origin.
- The sum of two even functions is even, and the sum of two odd functions is odd.
- The product of two even functions is even, and the product of two odd functions is even.
Definitions and Characteristics
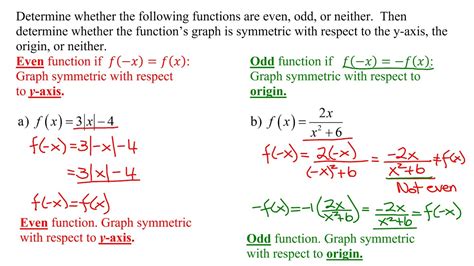
An even function is a function where f(x) = f(-x) for all x in its domain. This means that if we replace x with -x, the function remains unchanged. On the other hand, an odd function is a function where f(-x) = -f(x) for all x in its domain. This means that if we replace x with -x, the function changes sign.
Even functions have several key characteristics. They are symmetric with respect to the y-axis, meaning that if we reflect the function about the y-axis, we get the same function. They also satisfy the property f(x) + f(-x) = 2f(x) for all x. Examples of even functions include constant functions, quadratic functions, and cosine functions.
Odd functions, on the other hand, have different characteristics. They are symmetric with respect to the origin, meaning that if we rotate the function 180 degrees about the origin, we get the same function. They also satisfy the property f(-x) = -f(x) for all x. Examples of odd functions include linear functions, cubic functions, and sine functions.
Determining Whether a Function is Even or Odd
To determine whether a function is even or odd, we can follow these steps:
- Replace x with -x in the function.
- Simplify the resulting expression.
- Compare the simplified expression with the original function.
- If the two expressions are equal, the function is even.
- If the two expressions are opposites, the function is odd.
For example, let's consider the function f(x) = x^2. If we replace x with -x, we get f(-x) = (-x)^2 = x^2. Since f(x) = f(-x), we conclude that f(x) = x^2 is an even function.
Another example is the function f(x) = x^3. If we replace x with -x, we get f(-x) = (-x)^3 = -x^3. Since f(-x) = -f(x), we conclude that f(x) = x^3 is an odd function.
| Function | Even or Odd |
|---|---|
| f(x) = x^2 | Even |
| f(x) = x^3 | Odd |
| f(x) = sin(x) | Odd |
| f(x) = cos(x) | Even |

Real-World Applications

Even and odd functions have numerous real-world applications in physics, engineering, and computer science. For example, even functions are used to model symmetric phenomena, such as the vibration of a guitar string or the motion of a pendulum. Odd functions, on the other hand, are used to model asymmetric phenomena, such as the rotation of a wheel or the flow of a fluid.
In signal processing, even and odd functions are used to analyze and filter signals. Even functions are used to extract the symmetric components of a signal, while odd functions are used to extract the asymmetric components.
Common Mistakes and Limitations
When determining whether a function is even or odd, there are several common mistakes to avoid. One mistake is to assume that a function is even or odd based on its graph alone. While the graph of a function can provide visual cues about its symmetry, it’s essential to verify the function’s parity using algebraic methods.
Another limitation is that some functions may not be strictly even or odd. In such cases, we may need to use more advanced techniques, such as Fourier analysis, to decompose the function into its even and odd components.
What is the difference between an even and an odd function?
+An even function satisfies the property f(x) = f(-x) for all x, while an odd function satisfies the property f(-x) = -f(x) for all x.
How can I determine whether a function is even or odd?
+To determine whether a function is even or odd, replace x with -x in the function and simplify the resulting expression. Compare the simplified expression with the original function. If they are equal, the function is even. If they are opposites, the function is odd.
What are some common examples of even and odd functions?
+Common examples of even functions include constant functions, quadratic functions, and cosine functions. Common examples of odd functions include linear functions, cubic functions, and sine functions.
In conclusion, determining whether a function is even or odd is a crucial step in understanding its properties and behavior. By analyzing the function’s symmetry properties and using algebraic methods, we can quickly determine its parity. Even and odd functions have numerous real-world applications, and understanding their properties is essential for modeling and analyzing various phenomena in physics, engineering, and computer science.



