How To Find Hypotenuse
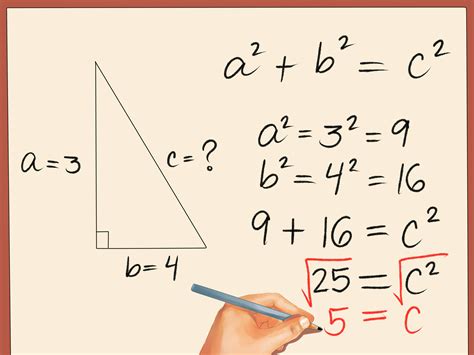
The concept of finding the hypotenuse of a right-angled triangle is a fundamental principle in geometry and trigonometry. The hypotenuse, which is the side opposite the right angle, can be calculated using the Pythagorean theorem. This theorem states that in a right-angled triangle, the square of the length of the hypotenuse (c) is equal to the sum of the squares of the lengths of the other two sides (a and b), or a^2 + b^2 = c^2.
Key Points
- The Pythagorean theorem is used to find the hypotenuse of a right-angled triangle.
- The formula for the Pythagorean theorem is a^2 + b^2 = c^2, where a and b are the lengths of the two shorter sides, and c is the length of the hypotenuse.
- This theorem can be applied in various real-world scenarios, such as construction, physics, and engineering.
- Understanding the concept of the hypotenuse and how to calculate it is essential for solving problems in geometry and trigonometry.
- There are also other methods to find the hypotenuse, including using trigonometric ratios such as sine, cosine, and tangent, though the Pythagorean theorem is the most direct method for right-angled triangles.
Understanding the Pythagorean Theorem

The Pythagorean theorem is named after the ancient Greek philosopher and mathematician Pythagoras, who is credited with its discovery. Although it is not known if he was the first to discover it, his contribution to its widespread acceptance and use in mathematics is undeniable. The theorem is applicable to all right-angled triangles, making it a versatile tool in geometry and trigonometry.
Applying the Pythagorean Theorem
To apply the Pythagorean theorem, one must know the lengths of the two shorter sides (a and b) of the right-angled triangle. The lengths of these sides can be determined through various means, such as measurement or calculation using other geometric principles. Once the lengths of sides a and b are known, the length of the hypotenuse © can be calculated using the formula c = √(a^2 + b^2).
| Side Lengths | Calculation | Hypotenuse Length |
|---|---|---|
| a = 3, b = 4 | c = √(3^2 + 4^2) | c = √(9 + 16) = √25 = 5 |

Real-World Applications

The ability to find the hypotenuse of a right-angled triangle has numerous real-world applications. In construction, for example, understanding the relationships between the sides of triangles is essential for designing and building structures. In physics, the Pythagorean theorem is used to calculate distances and velocities in problems involving right-angled triangles. Engineers also rely heavily on this theorem in their designs, from bridges to electronic circuits.
Using Trigonometric Ratios
While the Pythagorean theorem provides a direct method for finding the hypotenuse, trigonometric ratios such as sine, cosine, and tangent can also be used, especially when the lengths of the sides are not known but the angles and one side are. These ratios are defined as the ratios of the lengths of the sides of a right triangle and are essential tools in trigonometry and its applications.
For instance, if the length of one side and the angle opposite to it are known, the length of the hypotenuse can be found using the sine ratio: sin(θ) = opposite side / hypotenuse. Rearranging this formula gives the hypotenuse = opposite side / sin(θ). Similar calculations can be performed using the cosine and tangent ratios, depending on the known side and angle.
What is the Pythagorean theorem used for?
+The Pythagorean theorem is used to find the length of the hypotenuse of a right-angled triangle when the lengths of the other two sides are known. It has numerous applications in geometry, trigonometry, construction, physics, and engineering.
Can the Pythagorean theorem be used for all types of triangles?
+No, the Pythagorean theorem is specifically applicable to right-angled triangles. For other types of triangles, such as obtuse or acute triangles, different formulas and methods are required to find the lengths of the sides.
What are some real-world applications of finding the hypotenuse?
+Finding the hypotenuse has applications in construction for designing and building structures, in physics for calculating distances and velocities, and in engineering for designing various systems and structures.
In conclusion, finding the hypotenuse of a right-angled triangle is a fundamental concept in mathematics with wide-ranging applications. The Pythagorean theorem provides a straightforward method for calculating the hypotenuse when the lengths of the other two sides are known. Understanding and applying this theorem, along with trigonometric ratios, equips individuals with a powerful tool for solving problems in geometry, trigonometry, and various real-world scenarios.