Log Of 1
The concept of the logarithm of 1 is a fundamental idea in mathematics, particularly in the realm of algebra and calculus. At its core, the logarithm of a number is the power to which another fixed number, the base, must be raised to produce that number. In the case of the logarithm of 1, we are looking for the power to which the base must be raised to produce 1. This concept is crucial for understanding various mathematical operations and is used extensively in science, engineering, and finance.
Understanding the Logarithm of 1

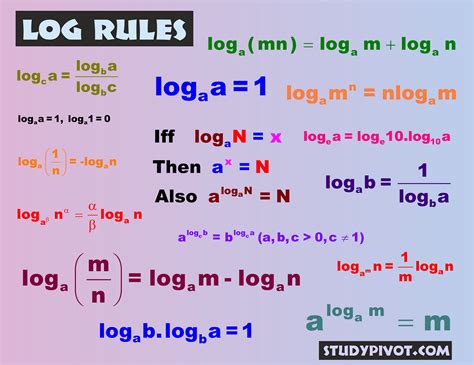
To delve deeper into the concept, it’s essential to understand that the logarithm of 1 is a special case. Regardless of the base used, the logarithm of 1 is always 0. This is because any number raised to the power of 0 equals 1. For example, in the logarithmic equation logb(1) = x, where b is the base, the solution x will always be 0, since b0 = 1 for any positive b not equal to 1. This property makes the logarithm of 1 a unique and straightforward case in logarithmic calculations.
Mathematical Representation and Properties
The mathematical representation of the logarithm of 1 can be expressed as logb(1) = 0, where b is the base of the logarithm. This property holds true for all bases except 1, as 1x = 1 for any x, which would lead to an undefined logarithm if the base were 1. Understanding this property is crucial for simplifying logarithmic expressions and solving equations involving logarithms.
| Base (b) | Logarithm of 1 (logb(1)) |
|---|---|
| Any positive b ≠ 1 | 0 |
| Example: Base 10 (log10(1)) | 0 |
| Example: Natural Logarithm (ln(1)) | 0 |
Key Points
- The logarithm of 1 is always 0, regardless of the base used, as any number raised to the power of 0 equals 1.
- This property is crucial for simplifying logarithmic expressions and solving logarithmic equations.
- The base of the logarithm cannot be 1, as this would result in an undefined mathematical operation.
- Understanding the logarithm of 1 is fundamental for advanced mathematical concepts, including calculus and algebra.
- The consistency of the logarithm of 1 across different bases makes it a reliable and essential component of mathematical and scientific calculations.
Applications and Implications

The concept of the logarithm of 1 has significant implications and applications across various fields. In science and engineering, logarithmic scales are often used to represent large ranges of values in a more manageable form. The fact that the logarithm of 1 is 0 provides a reference point on these scales, facilitating the comparison and analysis of data. Additionally, in calculus, the derivative of the natural logarithm function, which is 1/x, is used to model various phenomena, including population growth and chemical reactions. The logarithm of 1 serves as a foundational element in these calculations, ensuring the accuracy and reliability of the models.
Real-World Examples and Calculations
In real-world applications, understanding the logarithm of 1 can simplify complex calculations. For instance, when calculating the pH of a solution in chemistry, the formula pH = -log10([H+]) is used, where [H+] is the concentration of hydrogen ions. If the concentration of hydrogen ions is 1 (which would be a very acidic solution), the pH would be -log10(1) = 0, indicating an extremely acidic environment. This example illustrates how the logarithm of 1 can be applied in practical scenarios to derive meaningful conclusions.
Furthermore, the concept of the logarithm of 1 is not limited to mathematical and scientific applications. It also has implications in finance, particularly in the calculation of interest rates and investment returns. The formula for compound interest, A = P(1 + r/n)nt, where A is the amount of money accumulated after n years, including interest, P is the principal amount, r is the annual interest rate (in decimal), n is the number of times that interest is compounded per year, and t is the time the money is invested for in years, involves exponential growth. The logarithm of 1 plays a role in the analysis of such financial models, especially when considering the growth rate of investments over time.
What is the significance of the logarithm of 1 in mathematical calculations?
+The logarithm of 1 is significant because it provides a reference point for logarithmic scales and serves as a foundational element in various mathematical operations, including algebra and calculus. Its value, which is always 0 regardless of the base, simplifies complex calculations and is crucial for understanding and applying logarithmic properties.
How does the concept of the logarithm of 1 apply to real-world scenarios?
+The concept of the logarithm of 1 has practical applications in chemistry, finance, and other fields. For example, in chemistry, it's used in the calculation of pH levels, and in finance, it's relevant to the calculation of compound interest and investment returns. Understanding this concept can simplify complex calculations and provide insights into various phenomena.
Why is the base of the logarithm cannot be 1?
+The base of the logarithm cannot be 1 because 1x = 1 for any x, which would lead to an undefined logarithm. This is because the logarithmic function is defined as the inverse of the exponential function, and with a base of 1, this inverse relationship does not hold in a meaningful way, making the logarithm of 1 undefined for a base of 1.
In conclusion, the logarithm of 1 is a fundamental concept in mathematics, with far-reaching implications and applications across various fields. Its consistent value of 0, regardless of the base, makes it a reliable reference point for logarithmic calculations and a crucial element in understanding more complex mathematical concepts. As such, it remains an essential part of the foundation upon which more advanced mathematical theories and practical applications are built.



