Parabola Standard Form

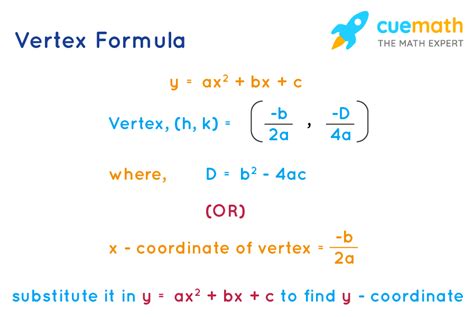
The parabola standard form is a fundamental concept in algebra and geometry, representing a U-shaped curve that opens upwards or downwards. This mathematical expression is crucial in understanding various phenomena in physics, engineering, and other fields. The standard form of a parabola is given by the equation $y = ax^2 + bx + c$, where $a$, $b$, and $c$ are constants, and $x$ and $y$ are the variables. The coefficient $a$ determines the direction and width of the parabola, with $a > 0$ indicating an upwards-opening parabola and $a < 0$ indicating a downwards-opening parabola.
Understanding the Parabola Standard Form

The parabola standard form can be rewritten as y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. This form is particularly useful for identifying the vertex and the axis of symmetry of the parabola. The axis of symmetry is given by the equation x = h, and it divides the parabola into two equal halves. The vertex form of a parabola is essential in solving problems related to quadratic equations and graphing parabolas.
Key Points
- The standard form of a parabola is given by $y = ax^2 + bx + c$.
- The coefficient $a$ determines the direction and width of the parabola.
- The vertex form of a parabola is $y = a(x - h)^2 + k$, where $(h, k)$ is the vertex.
- The axis of symmetry is given by $x = h$ and divides the parabola into two equal halves.
- Understanding the parabola standard form is crucial in solving quadratic equations and graphing parabolas.
Derivation of the Parabola Standard Form
The derivation of the parabola standard form involves completing the square of the quadratic equation y = ax^2 + bx + c. By rearranging the terms and adding and subtracting the square of half the coefficient of x, the equation can be rewritten as y = a(x - h)^2 + k. This process is essential in identifying the vertex and the axis of symmetry of the parabola. The derivation of the parabola standard form is a fundamental concept in algebra and is used extensively in solving quadratic equations and graphing parabolas.
| Parabola Standard Form | Vertex Form |
|---|---|
| $y = ax^2 + bx + c$ | $y = a(x - h)^2 + k$ |
| $a$ determines direction and width | $(h, k)$ is the vertex |
Applications of the Parabola Standard Form

The parabola standard form has numerous applications in physics, engineering, and other fields. In physics, parabolas are used to describe the trajectory of projectiles, the path of light rays, and the shape of mirrors and lenses. In engineering, parabolas are used in the design of bridges, arches, and other structures. The parabola standard form is also used in computer graphics, astronomy, and other fields. Understanding the parabola standard form is essential in solving problems related to quadratic equations and graphing parabolas.
Solving Quadratic Equations using the Parabola Standard Form
Solving quadratic equations using the parabola standard form involves rewriting the equation in the vertex form and then using the vertex to find the solutions. The vertex form of a parabola provides valuable information about the vertex and the axis of symmetry, making it easier to solve quadratic equations. The parabola standard form is also used in solving systems of equations and inequalities. Understanding the parabola standard form is crucial in solving problems related to quadratic equations and graphing parabolas.
What is the standard form of a parabola?
+The standard form of a parabola is given by $y = ax^2 + bx + c$.
What is the vertex form of a parabola?
+The vertex form of a parabola is given by $y = a(x - h)^2 + k$, where $(h, k)$ is the vertex.
What is the axis of symmetry of a parabola?
+The axis of symmetry of a parabola is given by $x = h$ and divides the parabola into two equal halves.
In conclusion, the parabola standard form is a fundamental concept in algebra and geometry, representing a U-shaped curve that opens upwards or downwards. Understanding the parabola standard form is essential in solving quadratic equations and graphing parabolas. The vertex form of a parabola provides valuable information about the vertex and the axis of symmetry, making it a crucial concept in algebra and geometry. The parabola standard form has numerous applications in physics, engineering, and other fields, making it a vital concept in various disciplines.