Rationalizing The Denominator

Rationalizing the denominator is a fundamental concept in algebra, particularly in the context of fractions and radicals. The process involves removing any radical from the denominator of a fraction, resulting in an equivalent expression with a rational denominator. This technique is essential in various mathematical operations, such as simplifying expressions, adding or subtracting fractions, and solving equations. In this article, we will delve into the principles and methods of rationalizing the denominator, exploring its significance and applications in mathematics.
Key Points
- Rationalizing the denominator involves removing radicals from the denominator of a fraction.
- The process is crucial for simplifying expressions, adding or subtracting fractions, and solving equations.
- There are two primary methods for rationalizing the denominator: multiplying by the conjugate and using the difference of squares.
- Rationalizing the denominator has numerous applications in mathematics, including algebra, geometry, and calculus.
- Understanding and mastering this concept is essential for problem-solving and critical thinking in mathematics.
Principles of Rationalizing the Denominator
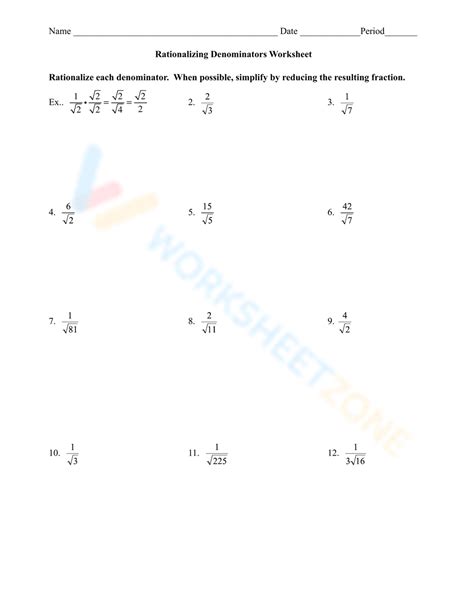
The primary goal of rationalizing the denominator is to eliminate any radical from the denominator, thereby obtaining an equivalent expression with a rational denominator. This is achieved by multiplying both the numerator and the denominator by a carefully chosen value, which removes the radical from the denominator. The chosen value is often the conjugate of the denominator or a cleverly selected expression that, when multiplied, results in a difference of squares.
Method 1: Multiplying by the Conjugate
One of the most common methods for rationalizing the denominator involves multiplying both the numerator and the denominator by the conjugate of the denominator. The conjugate of a binomial expression a + b is a - b, and vice versa. By multiplying the fraction by the conjugate of the denominator over itself, we can eliminate the radical from the denominator. This method is particularly useful when dealing with fractions containing square roots or other radicals.
| Expression | Conjugate | Rationalized Form |
|---|---|---|
| $\frac{1}{\sqrt{2} + 1}$ | $\sqrt{2} - 1$ | $\frac{\sqrt{2} - 1}{(\sqrt{2} + 1)(\sqrt{2} - 1)} = \frac{\sqrt{2} - 1}{2 - 1} = \sqrt{2} - 1$ |
| $\frac{1}{\sqrt{3} - 2}$ | $\sqrt{3} + 2$ | $\frac{\sqrt{3} + 2}{(\sqrt{3} - 2)(\sqrt{3} + 2)} = \frac{\sqrt{3} + 2}{3 - 4} = -\sqrt{3} - 2$ |

Method 2: Using the Difference of Squares
Another approach to rationalizing the denominator involves using the difference of squares identity: a^2 - b^2 = (a + b)(a - b). By recognizing that the denominator can be expressed as a difference of squares, we can multiply both the numerator and the denominator by the appropriate expression to remove the radical. This method is particularly useful when dealing with fractions containing higher-order radicals or complex expressions.
Applications and Significance

Rationalizing the denominator has far-reaching implications in various mathematical disciplines, including algebra, geometry, and calculus. The ability to simplify complex expressions and eliminate radicals from denominators is essential for solving equations, finding roots, and performing various mathematical operations. Moreover, rationalizing the denominator is a critical step in many mathematical proofs and derivations, demonstrating its fundamental importance in the field of mathematics.
In conclusion, rationalizing the denominator is a vital concept in algebra, enabling the simplification of complex expressions and the elimination of radicals from denominators. By mastering the principles and methods of rationalizing the denominator, individuals can develop a deeper understanding of mathematical operations and improve their problem-solving skills. Whether in algebra, geometry, or calculus, the ability to rationalize the denominator is an essential tool for any mathematician or student of mathematics.
What is the primary goal of rationalizing the denominator?
+The primary goal of rationalizing the denominator is to eliminate any radical from the denominator, resulting in an equivalent expression with a rational denominator.
What are the two primary methods for rationalizing the denominator?
+The two primary methods for rationalizing the denominator are multiplying by the conjugate and using the difference of squares.
Why is rationalizing the denominator important in mathematics?
+Rationalizing the denominator is important in mathematics because it enables the simplification of complex expressions, eliminates radicals from denominators, and facilitates various mathematical operations, such as solving equations and finding roots.