Reynolds Number Formula

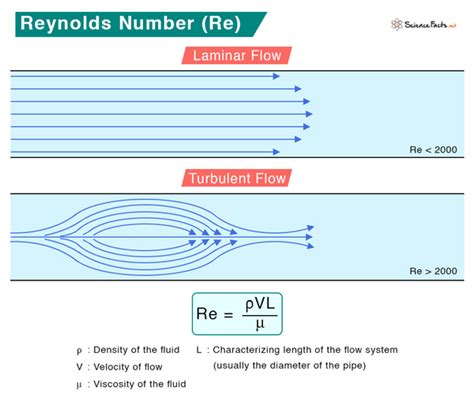
The Reynolds number is a dimensionless quantity used to predict flow patterns in different fluid flow situations. It is defined as the ratio of inertial forces to viscous forces and is used to distinguish between laminar and turbulent fluid flow. The Reynolds number formula is given by Re = ρUL/μ, where ρ is the fluid density, U is the flow velocity, L is the characteristic length, and μ is the dynamic viscosity of the fluid.
The Reynolds number is named after Osborne Reynolds, who first introduced the concept in the late 19th century. Reynolds conducted a series of experiments on fluid flow in pipes and observed that the flow pattern changed from laminar to turbulent as the flow velocity increased. He realized that the transition from laminar to turbulent flow was dependent on the ratio of inertial forces to viscous forces, which is now known as the Reynolds number.
Key Points
- The Reynolds number is a dimensionless quantity that predicts flow patterns in fluid flow situations.
- The formula for the Reynolds number is Re = ρUL/μ, where ρ is the fluid density, U is the flow velocity, L is the characteristic length, and μ is the dynamic viscosity of the fluid.
- The Reynolds number is used to distinguish between laminar and turbulent fluid flow.
- The transition from laminar to turbulent flow occurs at a critical Reynolds number, which is typically around 2000-4000 for pipe flow.
- The Reynolds number is an important parameter in fluid mechanics and is used in a wide range of applications, including pipe flow, boundary layer flow, and flow around objects.
Derivation of the Reynolds Number Formula
The Reynolds number formula can be derived by considering the forces acting on a fluid element in a flow situation. The inertial force acting on the fluid element is given by F_i = ρU^2, where ρ is the fluid density and U is the flow velocity. The viscous force acting on the fluid element is given by F_v = μU/L, where μ is the dynamic viscosity of the fluid and L is the characteristic length.
The Reynolds number is defined as the ratio of the inertial force to the viscous force, which is given by Re = F_i / F_v = ρU^2 / (μU/L) = ρUL/μ. This formula shows that the Reynolds number is a dimensionless quantity that depends on the fluid properties, flow velocity, and characteristic length.
Units and Dimensions of the Reynolds Number
The units of the Reynolds number are typically expressed in terms of the units of the fluid properties and flow parameters. For example, if the fluid density is expressed in units of kg/m^3, the flow velocity in units of m/s, the characteristic length in units of m, and the dynamic viscosity in units of Pa·s, then the Reynolds number is dimensionless and has no units.
The dimensions of the Reynolds number can be expressed in terms of the fundamental dimensions of mass, length, and time. The fluid density has dimensions of [M/L^3], the flow velocity has dimensions of [L/T], the characteristic length has dimensions of [L], and the dynamic viscosity has dimensions of [M/LT]. Substituting these dimensions into the Reynolds number formula, we get Re = ρUL/μ = ([M/L^3] \* [L/T] \* [L]) / ([M/LT]) = [1], which shows that the Reynolds number is a dimensionless quantity.
| Fluid Property | Units | Dimensions |
|---|---|---|
| Fluid Density (ρ) | kg/m^3 | [M/L^3] |
| Flow Velocity (U) | m/s | [L/T] |
| Characteristic Length (L) | m | [L] |
| Dynamic Viscosity (μ) | Pa·s | [M/LT] |

Applications of the Reynolds Number

The Reynolds number has a wide range of applications in fluid mechanics, including pipe flow, boundary layer flow, and flow around objects. In pipe flow, the Reynolds number is used to predict the transition from laminar to turbulent flow, which is critical in designing efficient piping systems. In boundary layer flow, the Reynolds number is used to predict the thickness of the boundary layer and the skin friction coefficient. In flow around objects, the Reynolds number is used to predict the flow pattern and the drag coefficient.
The Reynolds number is also used in a wide range of industries, including aerospace, chemical, and petroleum engineering. In aerospace engineering, the Reynolds number is used to predict the flow pattern around aircraft and spacecraft. In chemical engineering, the Reynolds number is used to predict the flow pattern in chemical reactors and piping systems. In petroleum engineering, the Reynolds number is used to predict the flow pattern in oil and gas pipelines.
Limitations and Challenges of the Reynolds Number
While the Reynolds number is a powerful tool for predicting flow patterns, it has several limitations and challenges. One of the main limitations of the Reynolds number is that it is based on a simplification of the Navier-Stokes equations, which assumes that the flow is incompressible and Newtonian. In reality, many fluids exhibit non-Newtonian behavior, and the flow can be compressible, which can affect the accuracy of the Reynolds number.
Another challenge of the Reynolds number is that it is sensitive to the choice of characteristic length and flow velocity. In some cases, the characteristic length and flow velocity may not be well-defined, which can lead to uncertainty in the Reynolds number. Additionally, the Reynolds number is a local parameter, which means that it can vary significantly over the flow domain, making it challenging to predict the flow pattern accurately.
What is the Reynolds number, and how is it used in fluid mechanics?
+The Reynolds number is a dimensionless quantity that predicts flow patterns in fluid flow situations. It is used to distinguish between laminar and turbulent fluid flow and is a critical parameter in designing and optimizing fluid flow systems.
How is the Reynolds number formula derived, and what are its units and dimensions?
+The Reynolds number formula is derived by considering the forces acting on a fluid element in a flow situation. The formula is Re = ρUL/μ, where ρ is the fluid density, U is the flow velocity, L is the characteristic length, and μ is the dynamic viscosity of the fluid. The units of the Reynolds number are typically expressed in terms of the units of the fluid properties and flow parameters, and its dimensions are [1], making it a dimensionless quantity.
What are some of the applications and limitations of the Reynolds number in fluid mechanics?
+The Reynolds number has a wide range of applications in fluid mechanics, including pipe flow, boundary layer flow, and flow around objects. However, it has several limitations, including its sensitivity to the choice of characteristic length and flow velocity, and its assumption of incompressible and Newtonian flow. Additionally, the Reynolds number is a local parameter, which can make it challenging to predict the flow pattern accurately.



