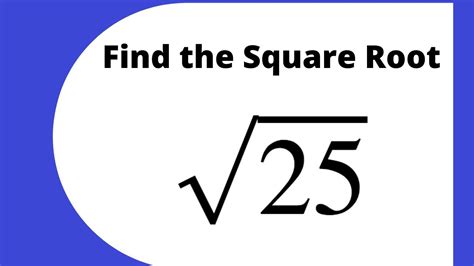
Square Root Of 25
The square root of a number is a value that, when multiplied by itself, gives the original number. In the case of 25, we are looking for a number that, when squared, equals 25. This is a fundamental concept in mathematics, particularly in algebra and geometry, where understanding square roots is crucial for solving equations and calculating distances and sizes of various shapes.
Calculating the Square Root of 25

To find the square root of 25, we look for a number that, when multiplied by itself, equals 25. This can be expressed as: √25 = x, where x is the number we are trying to find, and x * x = 25. By simple arithmetic, we know that 5 * 5 = 25, and -5 * -5 also equals 25, because a negative number multiplied by a negative number results in a positive number.
Understanding Positive and Negative Square Roots
In mathematics, every positive number has two square roots, one positive and one negative, except for zero, which has only one square root, zero itself. Therefore, the square roots of 25 are 5 and -5. This is an important concept to grasp because it affects how we solve equations and interpret results in various mathematical contexts.
| Number | Square Root |
|---|---|
| 25 | 5 and -5 |

Key Points
- The square root of a number is a value that, when multiplied by itself, gives the original number.
- The square root of 25 is 5 and -5, because both 5 * 5 and -5 * -5 equal 25.
- Every positive number has two square roots, one positive and one negative, except for zero.
- Understanding square roots is crucial for solving quadratic equations and has practical applications in physics, engineering, and computer science.
- The calculation of square roots can be applied to solve problems involving areas, volumes, and distances in geometric shapes.
Practical Applications of Square Roots

Square roots have numerous practical applications across various fields. For instance, in physics, the square root of the mass of an object can be used in calculations involving gravity and potential energy. In engineering, square roots are used in the design of bridges, buildings, and electronic circuits. The ability to calculate and understand square roots is thus a fundamental skill for professionals in these fields.
Calculating Square Roots of Other Numbers
Beyond the square root of 25, calculating the square roots of other numbers can be more complex, especially when dealing with numbers that are not perfect squares. For these cases, mathematicians use various methods, including the long division method, the Babylonian method, or calculators and computer software for more precise calculations.
In conclusion, understanding the concept of square roots, including the square root of 25, is essential for advancing in mathematics and applying mathematical principles to real-world problems. The square roots of 25, which are 5 and -5, illustrate the basic principle that every positive number has two square roots, a concept that has far-reaching implications in science, technology, engineering, and mathematics (STEM) fields.
What is the square root of a number?
+The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 25 is 5, because 5 * 5 = 25.
How do you calculate the square root of a number that is not a perfect square?
+For numbers that are not perfect squares, various methods can be used, including the long division method, the Babylonian method, or using calculators and computer software for more precise calculations.
What are the practical applications of understanding square roots?
+Understanding square roots has practical applications in physics, engineering, and computer science, including solving problems related to distances, velocities, frequencies, and designing geometric shapes and structures.