Symmetric Property Of Equality
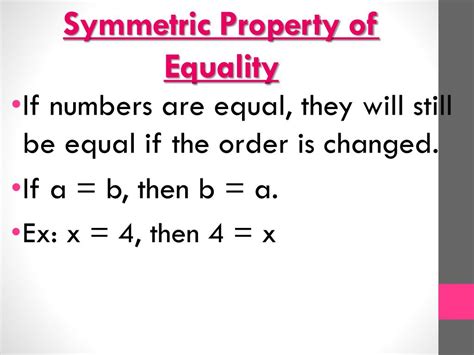
The symmetric property of equality is a fundamental concept in mathematics that states if a equals b, then b equals a. This property is often denoted as: if a = b, then b = a. It is one of the three properties of equality, alongside the reflexive and transitive properties, which together form the backbone of algebraic manipulations and mathematical reasoning.
Understanding the Symmetric Property

The symmetric property essentially implies that the order of the elements in an equation does not change the truth of the equation. It is a basic principle that allows for the manipulation of equations to solve for unknowns and to simplify expressions. For instance, if we know that 2x + 5 = 11, we can use the symmetric property to rewrite this equation as 11 = 2x + 5, which does not change the solution set for x.
Importance in Algebraic Manipulations
In algebra, the symmetric property is crucial for solving equations and inequalities. It enables the rearrangement of terms in an equation, which is essential for isolating the variable. For example, starting with x + 3 = 7, applying the symmetric property allows us to rewrite it as 7 = x + 3, and then, through further manipulations, solve for x.| Initial Equation | Symmetric Form |
|---|---|
| $x + 3 = 7$ | $7 = x + 3$ |
| $2x - 4 = 12$ | $12 = 2x - 4$ |

Applications in Mathematics and Beyond

The symmetric property of equality has far-reaching implications beyond basic algebra. It plays a critical role in various areas of mathematics, including geometry, where it is used to establish the congruence of figures, and in calculus, where it aids in the manipulation of limits and derivatives.
Geometric Applications
In geometry, the symmetric property is integral to the concept of congruent figures. Two figures are said to be congruent if one can be transformed into the other through a combination of translations, rotations, and reflections. The symmetric property ensures that if figure A is congruent to figure B, then figure B is also congruent to figure A, which is crucial for proving geometric theorems and properties.The symmetric property also has practical applications in fields like physics and engineering, where the balance and symmetry of structures are critical for stability and efficiency. Understanding and applying the symmetric property can help in designing and analyzing systems that require equilibrium, such as bridges and buildings.
Key Points
- The symmetric property of equality states that if $a = b$, then $b = a$.
- It is essential for algebraic manipulations, allowing for the rearrangement of equations to solve for unknowns.
- The property has applications in geometry, particularly in establishing the congruence of figures.
- It plays a role in various mathematical disciplines, including calculus, and has practical applications in physics and engineering.
- Understanding the symmetric property is crucial for appreciating the concept of equivalence and two-way relationships in mathematics.
Conclusion and Future Directions
The symmetric property of equality is a foundational concept in mathematics, underpinning many of the algebraic manipulations and geometric principles that are taught in schools and used in professional fields. Its implications extend beyond the classroom, influencing how we design, analyze, and solve problems in a wide range of disciplines. As mathematics continues to evolve, understanding and applying the symmetric property will remain essential for advancing knowledge and solving complex problems.What is the symmetric property of equality?
+The symmetric property of equality states that if a = b, then b = a. It means that the order of the elements in an equation does not change the truth of the equation.
Why is the symmetric property important in algebra?
+The symmetric property is crucial for solving equations and inequalities. It allows for the rearrangement of terms, which is essential for isolating the variable and solving for unknowns.
Are there applications of the symmetric property beyond mathematics?
+Yes, the symmetric property has practical applications in fields like physics and engineering, particularly in designing and analyzing systems that require balance and symmetry for stability and efficiency.



