Volume Of A Hemisphere

The volume of a hemisphere is a fundamental concept in geometry, and it has numerous applications in various fields, including architecture, engineering, and physics. A hemisphere is half of a sphere, and its volume can be calculated using a simple formula. To understand the volume of a hemisphere, it's essential to start with the basics of spherical geometry and then delve into the calculations involved.
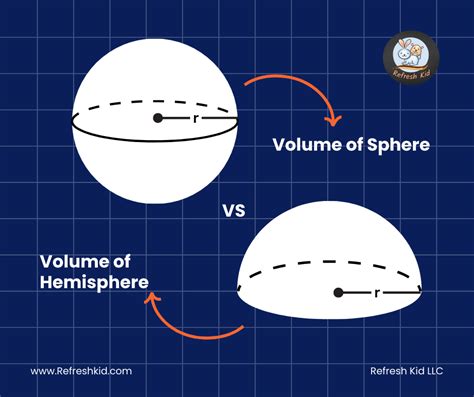
In geometry, a sphere is a three-dimensional shape that is perfectly symmetrical about its center. The volume of a sphere (V) can be calculated using the formula V = (4/3)πr³, where r is the radius of the sphere. When a sphere is divided into two equal halves, each half is called a hemisphere. The volume of a hemisphere is, therefore, half the volume of a full sphere. This can be calculated using the formula V = (2/3)πr³, where r is the radius of the hemisphere.
Key Points
- The volume of a hemisphere is half the volume of a full sphere.
- The formula to calculate the volume of a hemisphere is V = (2/3)πr³.
- The volume of a hemisphere is directly proportional to the cube of its radius.
- Understanding the volume of a hemisphere is crucial in various applications, including architecture and engineering.
- The formula for the volume of a hemisphere can be derived from the formula for the volume of a sphere.
Derivation of the Formula

The formula for the volume of a hemisphere can be derived from the formula for the volume of a sphere. As mentioned earlier, the volume of a sphere is given by V = (4⁄3)πr³. Since a hemisphere is half of a sphere, its volume can be calculated by simply dividing the volume of the sphere by 2. Therefore, the volume of a hemisphere (V) is V = (1⁄2) * (4⁄3)πr³, which simplifies to V = (2⁄3)πr³.
Calculating the Volume
To calculate the volume of a hemisphere, you need to know the radius of the hemisphere. Once you have the radius, you can plug it into the formula V = (2⁄3)πr³. For example, if the radius of the hemisphere is 5 cm, the volume can be calculated as V = (2⁄3)π(5)³. Using the value of π as approximately 3.14159, the calculation becomes V = (2⁄3) * 3.14159 * 125, which equals approximately 261.799 cm³.
| Radius of Hemisphere | Volume of Hemisphere |
|---|---|
| 5 cm | approximately 261.799 cm³ |
| 10 cm | approximately 2094.4 cm³ |
| 15 cm | approximately 7065.59 cm³ |
Applications and Importance

The concept of the volume of a hemisphere has numerous practical applications. In architecture, for instance, understanding the volume of hemispherical structures is crucial for designing domes, vaults, and other curved features of buildings. In engineering, the volume of a hemisphere is important in the design of tanks, reservoirs, and other containers that may have hemispherical ends. Additionally, in physics, the volume of a hemisphere is used in calculations involving the buoyancy of objects partially submerged in fluids.
Real-World Examples
Real-world examples of hemispherical structures include the domes of many famous buildings, such as St. Peter’s Basilica in Vatican City and the United States Capitol in Washington, D.C. These structures are not only aesthetically pleasing but also demonstrate a deep understanding of geometric principles, including the calculation of volumes of complex shapes like hemispheres.
In conclusion, the volume of a hemisphere is a fundamental geometric concept with wide-ranging applications. Understanding how to calculate the volume of a hemisphere and appreciating its importance in various fields can provide valuable insights into the intricate relationships between geometric shapes and their real-world applications.
What is the formula for calculating the volume of a hemisphere?
+The formula for the volume of a hemisphere is V = (2⁄3)πr³, where r is the radius of the hemisphere.
How is the volume of a hemisphere derived from the volume of a sphere?
+The volume of a hemisphere is derived by dividing the volume of a sphere by 2, since a hemisphere is half of a sphere. This results in the formula V = (2⁄3)πr³ from the sphere’s volume formula V = (4⁄3)πr³.
What are some real-world applications of understanding the volume of a hemisphere?
+Understanding the volume of a hemisphere has applications in architecture, engineering, and physics, including the design of domes, tanks, and other structures, as well as calculations involving buoyancy.